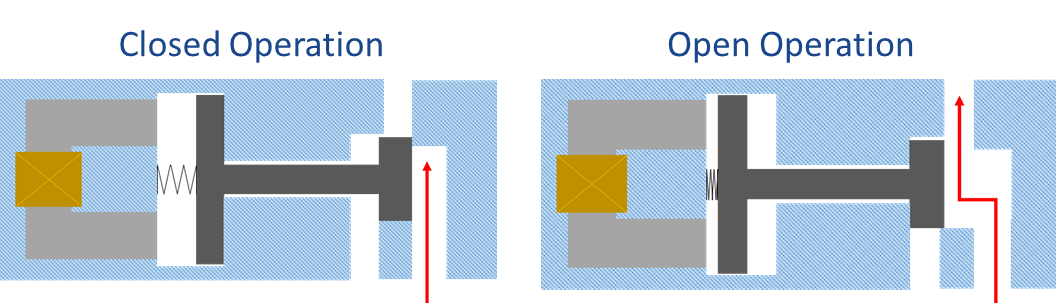
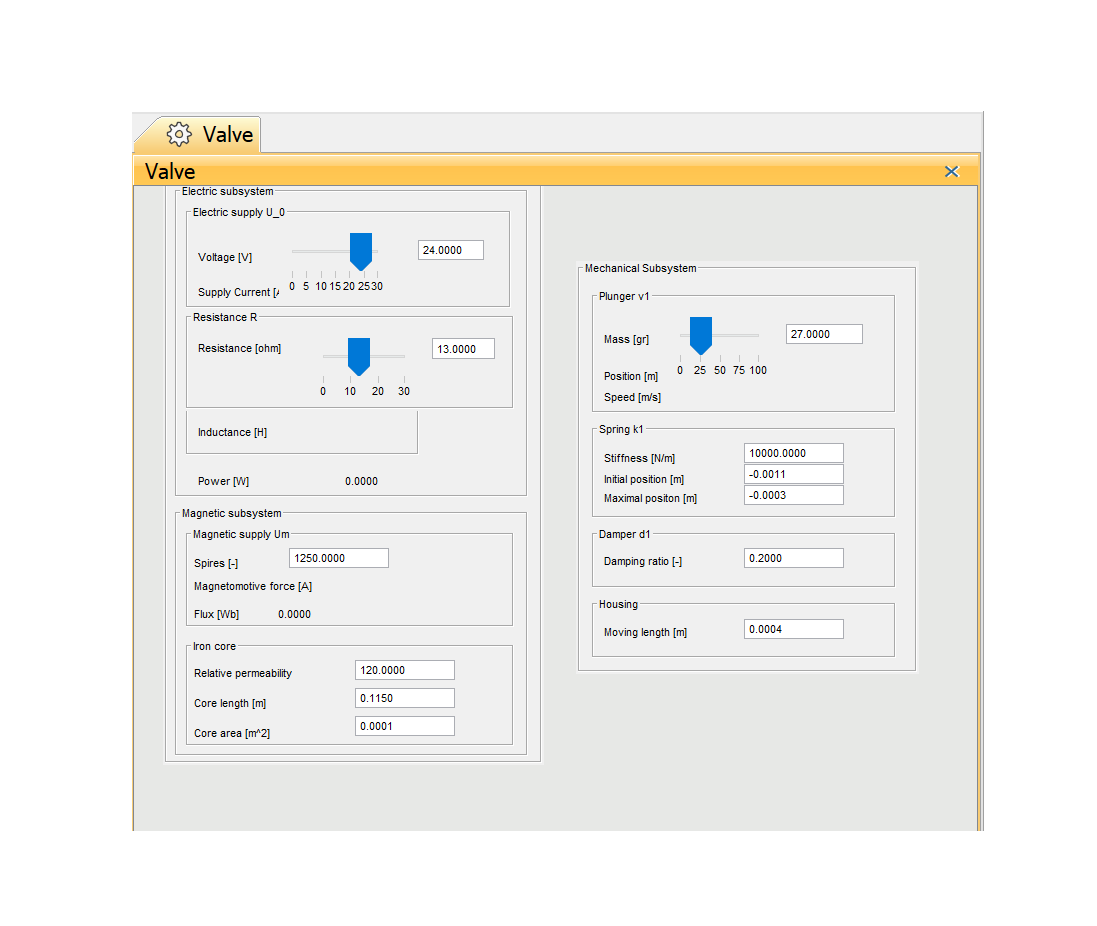
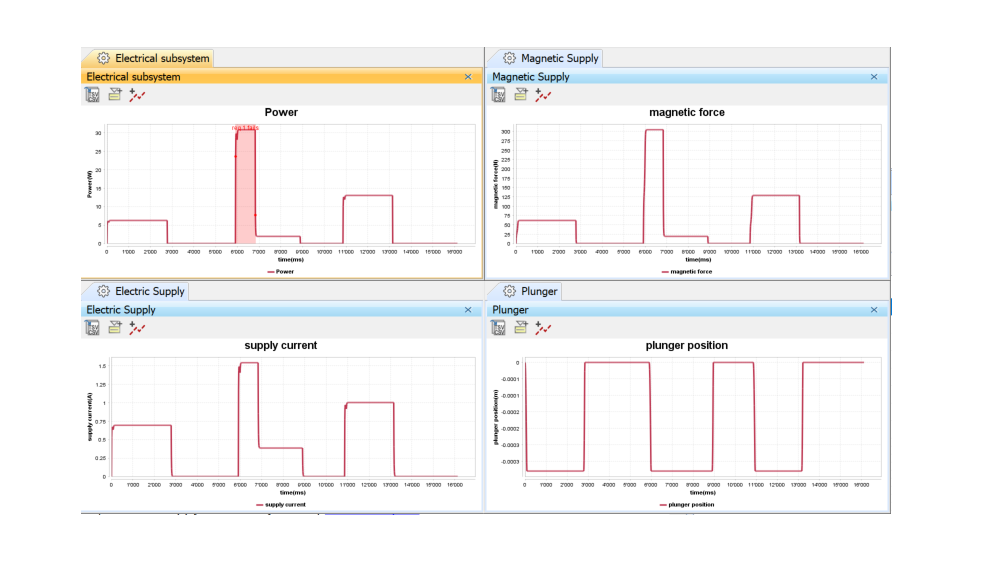
Electromechanical Valve Physical Model
This article presents an example of a valve model built by ROSAS' Model-Based Engineering department.
Expertise |
Model Based Engineering, Safety & Reliability |
|---|